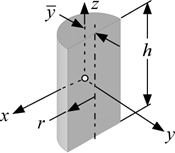
MOI_HalfCylinder

This procedure returns mass and moments of inertia of a half cylinder.
Inputs
r = radius of cylinder [m, ft]
h = length of cylinder [m, ft]
rho = material density [kg/m^3, lbm/ft^3]
Outputs
m=mass [kg, lb_m]
I_x = moment of inertia about the x-axis [kg-m^2 or lbm-ft^2]
I_y= moment of inertia about the y-axis [kg-m^2 or lbm-ft^2]
I_z = moment of inertia about the z-axis [kg-m^2 or lbm-ft^2]
y_bar = distance from centroid to flat surface [m or ft]
Example:
$Load Mechanical Design
$UnitSystem SI K Pa
$VarInfo I_x units=kg-m^2
$VarInfo I_y units=kg-m^2
$VarInfo I_z units=kg-m^2
r=0.2 [m]
h=0.4 [m]
rho=990 [kg/m^3]
Call moi_halfcylinder(r, h, rho: m, I_x, I_y, I_z, y_bar)
{Solution:
I_x=0.4013 [kg-m^2]
I_y=0.5806 [kg-m^2]
I_z=0.3184 [kg-m^2]
y_bar = 0.08488 [m]
m = 24.88 [kg]
}
Reference: Gray, G.L, F. Costanzo, R.J. Witt, and M.E. Plesha, Engineering Mechanics: Statics and Dynamics, Third Edition, McGraw Hill, (2023).